Simple harmonic motion is a periodic motion in which a particle move to and fro repeatedly about a mean position in presence of restoring force. Mean position is the central position where particle’s displacement is zero or where particle is at equilibrium position. Examples of shm plays important roles in our daily life as well as understanding different physical phenomenons.
What is amplitude in SHM?
Amplitude is the displacement of particle from it’s mean position or equilibrium position. In harmonic motion, amplitude is always directed away from mean position. All periodic motion exhibiting harmonic motion is due to force called restoring force. This restoring force is directly proportional to its amplitude (displacement).
F ∝ -y
or, F = -ky
Where,
F = Restoring force
k = force constant
y = displacement of particles
Negative sign (-ve) represents that restoring force is always directed towards equilibrium position.
Variation of quantities on variation of amplitude
There is variation of quantities like displacement, velocity, acceleration, K.E and P.E of systems exhibiting S.H.M and this variation is due to variation of amplitude at different point of harmonic motion. So, this changes at mean and extreme position can be tabulated as below.
At mean position the value of amplitude is zero but at extreme position its value is equal to the displacement covered (r=y).
| Quantity | At Mean Position | At Extreme Position |
|---|---|---|
| Dsplacement | y = 0 (min) | y = r (max) |
| Velocity | v = rω | v = 0 |
| Acceleration | a = 0 | a= ω2r |
| Kinetic Energy | K.E = 1/2 mω2r2 | K.E = 0 |
| Potential Energy | P.E = 0 | P.E = 1/2 mω2r2 |
| Total Energy | T.E = constant | T.E = constant |
Amplitude and time period of pendulum
Time period of simple pendulum is given by
T = 2π√l/g
From above equation,
It is clear that time period of pendulum is independent of amplitude, mass and material of oscillating body.
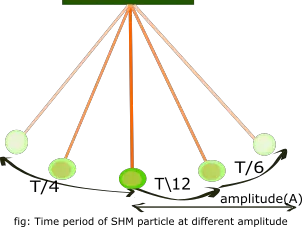
Time calculation at different amplitude
Let us suppose a particle/body oscillating SHM with an amplitude ‘r’ and time period T. Then time taken to travel different amplitude/displacement can be calculated as related as:
Case I
Time taken to travel A/2 amplitude from mean position
From equation of SHM,
We have
y = rsinωt
or, A/2 = rsinωt
or, 1/2 = sinωt
or, ωt = sin-1 (1/2)
or, 2π/T . t = 30°
or, 2π/T . t = π/6
t = T/12
Thus, Time taken to travel A/2 amplitude from mean position is T/12.
Case II
Time taken to travel A/2 amplitude from extreme position is T/6.
Case III
Time taken to travel A amplitude from mean position or from extreme position to mean position is T/4 .
Quick Facts
- All particle executing SHM are periodic motion but all periodic motion are not SHM.
- Equation of SHM starting from mean position is y = rsinωt
- Equation of SHM starting from other point but not from mean position is y = rsin(ωt ± φ)
- Equation of simple harmonic motion starting from extreme position is y = rcosωt (φ = 90°).
References